While I was looking for a gift for a child’s birthday, a math book fell into my hands. I am always fascinated when authors write about abstract scientific topics for children, whether it’s on Albert Einstein’s theories, the life of Marie Curie, technology or space travel. But this particular book was different. It’s all about prime numbers—specifically twin primes. Danish author Jan Egesborg has endeavored to introduce children to one of the most stubborn open problems in number theory, which even the brightest minds have repeatedly failed to solve over the past 100-plus years: the twin prime conjecture.
As is so often the case in mathematics, the conjecture falls into the category of those that are easy to understand but devilishly hard to prove. Twin primes are two prime numbers that have a distance of two on the number line; that is, they are directly consecutive if you ignore even numbers. Examples include 3 and 5, 5 and 7, and 17 and 19. You can find a lot of twin primes among small numbers, but the farther up the number line you go, the rarer they become.
That’s no surprise, given that prime numbers are increasingly rare among large numbers. Nevertheless, people have known since ancient times that infinitely many prime numbers exist, and the prime number twin conjecture states that there are an infinite number of prime number twins, as well. That would mean that no matter how large the values considered, there will always be prime numbers in direct succession among the odd numbers.
On supporting science journalism
If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
Admittedly, translating these concepts for kids is not easy (which is why I have so much respect for Egesborg and his children’s book). Prime numbers (2, 3, 5, 7, 11, 13,…) are like the fundamental particles of the natural numbers. They are only divisible by 1 and themselves. All other natural numbers can be broken down into their prime divisors, which makes prime numbers the basic building blocks of the mathematical world.
A Proof from Antiquity
Mathematics has an unlimited number of prime number building blocks. Euclid proved this more than 2,000 years ago with a simple thought experiment. Suppose there were only a finite number of prime numbers, the largest being p. In this case, all prime numbers up to p could be multiplied together.
In this case, you could multiply all prime numbers up to p with each other and add 1: 2 x 3 x 5 x 7 x 11 x … x p + 1. The result cannot be divided by any of the existing prime numbers. This means that the number 2 x 3 x 5 x 7 x 11 x … x p + 1 is either prime or has a prime factor that does not appear in the original 2, 3,…, p primes. Therefore, no finite list of primes can ever be complete; it will always be possible to construct additional ones. It follows that there are infinitely many prime numbers.
Not all mysteries about prime numbers have been solved, however. Their distribution on the number line, in particular, remains a mystery. Although we know that prime numbers appear less and less frequently among large numbers, it is not possible to specify exactly how they are distributed.
In principle, the average distance between one prime number and the next is the value ln(p). For the small number p =19, this corresponds to ln(19) ≈ 3. For the large prime number 2,147,483,647, the distance is around 22. For the huge value 531,137,992,816,767,098,689,588,206,552,468,627,329,593,117,727,031,923,199,444,138,200,403,559,860,852,242,739,162,502,265,229,285,668,889,329,486,246,501,015,346,579,337,652,707,239,409,519,978,766,587,351,943,831,270,835,393,219,031,728,127 (also a prime number), the distance is around 420.
As these examples illustrate, the average distance between the prime numbers increases with the size of p. And this fact makes prime number twins, which have the smallest possible distance between them (apart from 2 and 3), so interesting to number theorists. As the average distance between prime numbers increases, it could be that at a certain point there are no more twins. Yet most experts think otherwise. Why, they reason, should there be a certain point on the number line from which no more twin primes suddenly appear? What makes this one point so special? Number theorists assume that even if these prime number twins become rarer, you will always eventually come across another pair.
Computer calculations to date seem to support this view. The largest pair of prime number twins found so far is: 2,996,863,034,895 x 21,290,000 + 1 and 2,996,863,034,895 x 21,290,000 – 1, both numbers with 388,342 digits. A computer-aided search will never be able to prove that there are an infinite number of twin primes, however. Stronger tactics are needed.
An Unexpected Surprise
A little-known mathematician delivered just that in 2013. Yitang Zhang had previously been a household name among very few specialists—but then he published a paper that hit the number theory world like a bomb. He was not able to prove the prime number twin conjecture but demonstrated something close to it, which was more progress than anyone had made since the twin prime conjecture was formulated in the 19th century.
Zhang showed that there are an infinite number of pairs of prime numbers of the type (p, p + N) with a distance N between them that is less than 70 million. The twin prime conjecture would have been proved if he had been able to prove his result for N=2. Instead Zhang demonstrated that among all pairs of prime numbers with a distance of less than 70 million, there is at least one pairing (p, p + N) that occurs infinitely often.
This proof was a huge step forward because mathematicians are not only interested in prime number twins but also in other types of prime number pairs, such as those with a distance of four (such as 3 and 7 or 19 and 23), the so-called cousin primes, or those with a distance of six (such as 5 and 11 or 11 and 17), the so-called sexy primes. In general, it is unclear whether an infinite number of any of these pairings exist.
Zhang achieved this astonishing result using what mathematicians call prime number sieves. These constructs can be imagined as a real sieve: you tip all the natural numbers into it and filter out all the values that are not prime numbers. This idea is named for the ancient Greek scholar and mathematician Eratosthenes, though the first known written record of it is from a few centuries after he lived. It involves a list of natural numbers in which one removes every even value (apart from 2), then all multiples of 3, multiples of 5, and so on, such that only the prime numbers remain at the end.
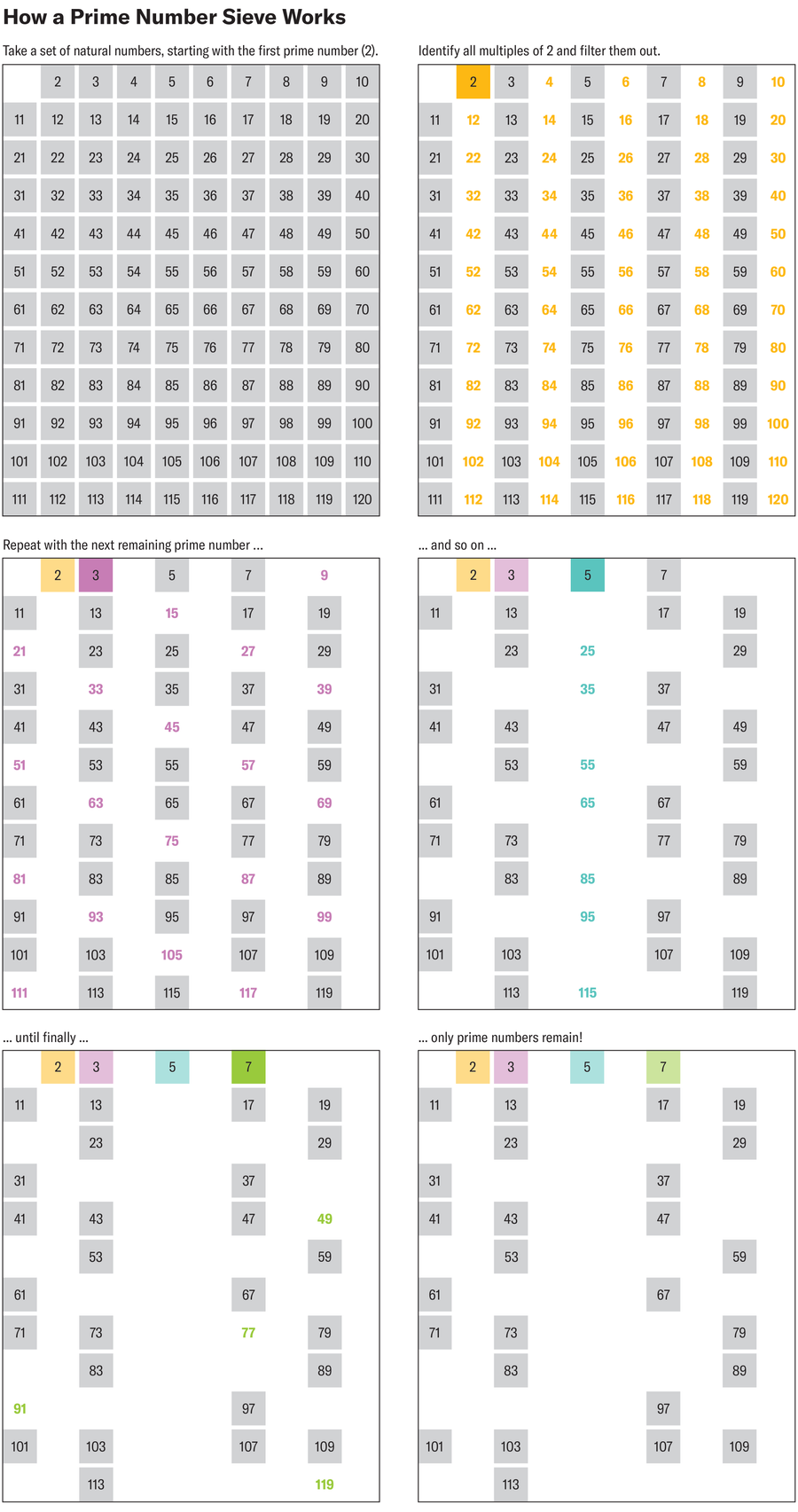
By going through all the natural numbers one by one and eliminating their multiples (except for the number itself), only prime numbers will remain.
Amanda Montañez
Although the sieve of Eratosthenes is exact, it is very difficult to apply to concrete problems from a mathematical point of view. Using this method to prove general statements about prime numbers seems hopeless in most cases. Zhang therefore turned to another sieve that only sifts out numbers with large prime divisors. Although this sieve is not as effective as others, it allows enough flexibility to carry out extensive proofs. Zhang worked single-handedly on the twin prime conjecture for years—number theory was not actually part of his research area.
This persistence paid off: Zhang proved that there is at least one kind of prime number pair with a distance of less than 70 million that occurs infinitely often. And the next breakthrough was not long in coming.
Number theorists from all over the world pounced on Zhang’s result and tried to improve it. A joint project was set up, and numerous experts joined in. By optimizing Zhang’s method, they were able to reduce the maximum distance N between the pairs of prime numbers to get as close as possible to 2. Within a few months, they showed that there is at least one type of prime number pair with a maximum distance of 4,680 that occurs infinitely often. Around the same time, two Fields Medalists, Terence Tao and James Maynard, independently developed a modified sieve that enabled them to reduce the result to 246, an unbroken record to date.
In concrete terms, this means that if you look at all pairs of prime numbers (p, p + N) that have a distance between N=2 and N=246, then there is at least one such pair that occurs infinitely often. The sieving methods cannot be generalized so far as to push the result down to N=2, however.
Still, the results mark unexpected progress in an area that leaves many experts baffled. Maynard makes this clear in a Numberphile YouTube video: “This is one of the interesting and frustrating things about prime numbers: that often it’s clear what the right answer should be…. The game is always trying to rule out there being some very bizarre conspiracy among prime numbers that would mean that they would behave in a rather different way to how we believe that they should behave.”
Of course, Egesborg could not include all these details in his children’s book on the subject. Nevertheless, he managed to write a book that conveys a few mathematical concepts in a playful way.
I bought the book and gave it to the child in question on his birthday—and. his parents later told me that he had thoroughly enjoyed it. As I found out afterward, however, this was less a result of the mathematical content than the fact that a frog farts loudly on one of the first pages.
This article originally appeared in Spektrum der Wissenschaft and was reproduced with permission.
Note: This article have been indexed to our site. We do not claim legitimacy, ownership or copyright of any of the content above. To see the article at original source Click Here













